A fundamental Exploration of a parametric equation
(This was my first write-up done on Aug. 31, and Sep. 8, 2009,
in which I included some basic case analysis. )
Chen Tian
Here is the parametric equation we will try to explore in the
write-up.
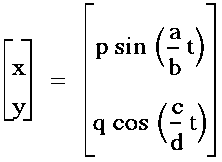with t in some range.
When
p, a, b, q, c, d are all equal to 1Õs,
1) We let t go from 0 to 1.57
(approximate pi/2), then we will see a quart of circle
in the first quadrant.
2) Similarly let t go from 0 to
3.14 (approximate pi), the semi-circle appears in the first and fourth
quadrant.
3) We directly let t go from 0
to 6.28 (approximate 2pi), a circle shows up in the
grid with the center at point (0,0).
Why
is this?
It
just shows the relation between values of sin(t) and cos(t),
which usually are shown in two separate ÒwaveÓ graphs of sin and cos.
If
p, a, b, q, c, d are all equal to 3Õs, obviously , the
circle becomes larger with radius of length 3.
But
how can we move the circle to wherever we want? After some trying, we find
adding a certain real number to the x value takes the circle to the right,
subtracting a certain real number to the x value takes the circle to the left,
similarly adding a certain real number to the y value takes the circle to the
up, subtracting a certain real number to the y value takes the circle to the
down, respectively, with the distance of the real number.
Interestingly,
isnÕt this another way to draw a circle with an
arbitrary radius centered at the origin or somewhere else in the coordinate
plan?
The
example of (x, y)=(3sin(t)+2.4, 3(cos(t)-0.3) with t from (0, 6.28) is shown in the below figure.
What
if p=2 , a, b, q, c, d are all equal to 1Õs? A horizontal ellipse at the origin will
be graphed like the following one.
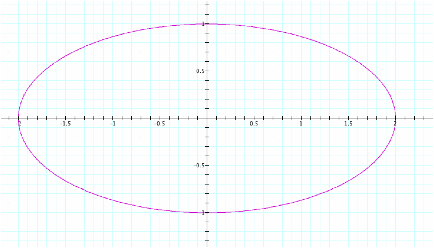
Why?
It is because the x values are double when y values keep as the same as in the
all 1Õs cases.
So
we can image that when q=2, p, a, b, c, d are all equal
to 1Õs, a vertical ellipse will be graphed. If q goes greater, the ellipse will look like being
ÒsqueezedÓ more.
From
now on, we move from arcs to circles then to ellipses. WasnÕt that pleasing?
What
if a, b, c, d are not all equal to 1Õs?
Keeping
the other parameters in the same hypothesis for the unit circle case, let b go greater.
When
b=1.01 goes to b=1.03, the unit circle I get a little bit slanted and there is
the little hole appearing around point (-0.2, 1); up to b=1.1, the hole gets
bigger, and the circle gets more slanted; then up to b=1.4, the unit circle
does not look like itself any more; then up to b=1.6, the curve looks like the
Greek letter gama; then up to around b= 1.9, the
space ÒinsideÓ the gama-like curve gets smaller, and
the curve looks being folded into two halves.
What
happened to the changes from the circle to the curve? When b gets greater, a/b
gets smaller, and the range of the values of sin((a/b)t)
gets changing, so it plots different sets of points (x,y).
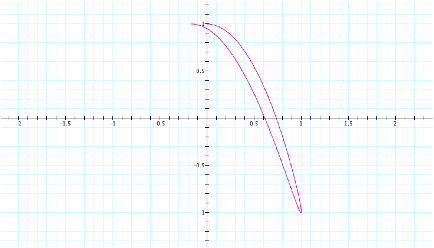
Notice,
when b=2, the Òtwo halvesÓ of the gama-like curve
coincide. It is just like the right half of a parabola.
Why?
(½)t is in the interval [0, 3.14],
sin((½)t) goes from 0 up to 1 and then down to 0 again, and cos(t) goes from 1 to -1 when t goes from 0 to pi, so the
points on the Òright half of a parabolaÓ curve does go back and forth once.
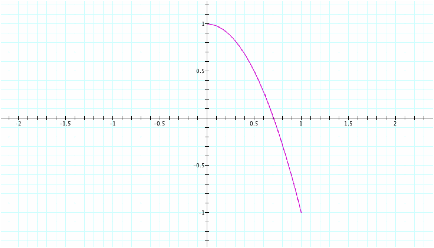
When
b=3 the curve looks Greek letter gama
again. When b=5 the right extremity of the gama-like
curve seems intending to Òpoint toward outsideÓ, which makes the curve looks
like the letter v. When b goes
greater from 5 to much greater number, say 80, the curve looks more like a
letter v, which gets thinner and thinner. When b gets greater and greater a/b
approaches to 0, the v-like curve gets extremely slim, but it will never
becomes a line. –- Well, for women who want to lose weight fast this might
be a better and cheaper product than Òslim-fastÓ sold in Wal
Mart.
What
all we have discussed above is confined with t in the interval [0, 6.28]. So
letÕs observe some more cases when t is in the interval [-6.28, 6.28]. Thus,
the domain of x values will be symmetric about the y axis.
Look
at the graph when b=1.9 compared with the one weÕve seen above. We will see
this new curve is the curve in the above b=1.9 graph plus its reflection about
the y axis.
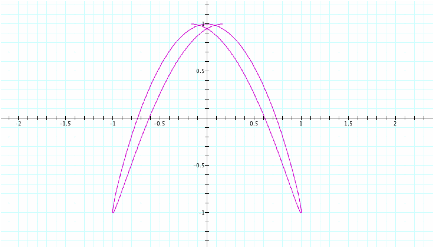
Therefore, we claim when
b=2 the curve will be a ÒcompleteÓ parabola comparing to the above curve of
Òright half of a parabolaÓ. Again, another way to draw a
parabola.
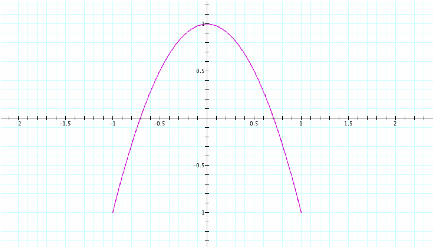
Therefore,
using the idea of reflection we will understand better why a curve looks like
what it is. Now letÕs snap some curves with some specific parameters as
follows.
b=1.07
b=1.5
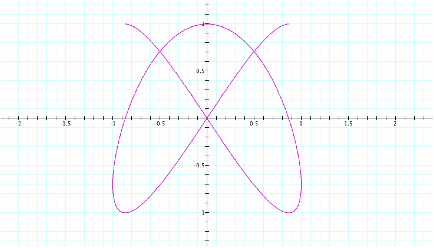
b=4
or b=-4
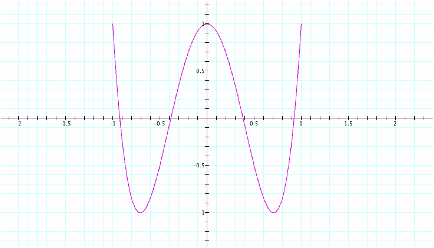
Ok,
now let observe a few more complex cases.
When
p, b, q, c, d are all equal to 1Õs,
if
a=2,
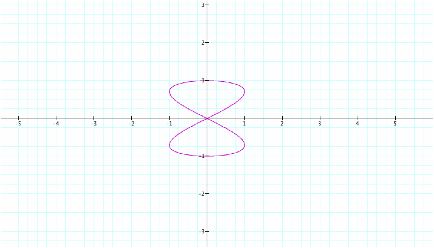
if
a=3
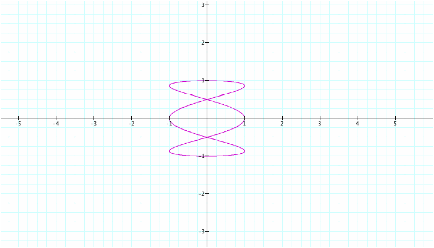
if
a=4
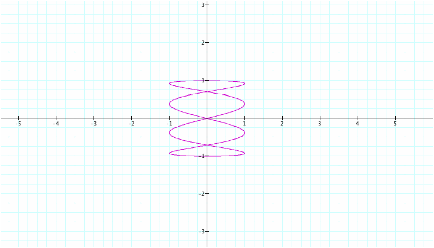
if
a=5
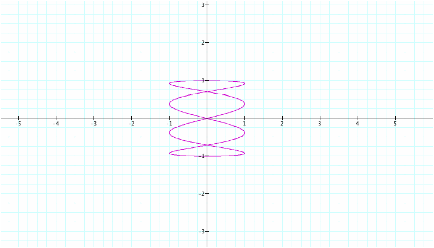
if
a=8
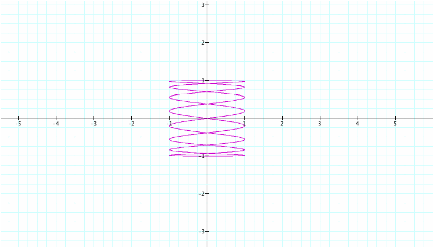
So,
it seems when the number of the ÒloopsÓ formed by the curve equals the value of
a. But, why?
One thing I can say is that when a gets greater, the value of sin(at) goes back and forth faster, i.e., the frequency of
the ÒvibrationÓ of the Òcombination waveÓ of sin and cos
gets bigger. As for the corresponding equality relationship between number of
the ÒloopsÓ and the value of a when (x, y)=(sin(at), cos(t)) for t in [0, 6.28], my explanation would be that
when a=1 the value of sin(at) goes through a Òvibration cycleÓ
(maybe namely, ÒperiodÓ); when a=2, the speed of the change of the value of sin(at) is doubled, so the value of sin(at)
goes through two Òvibration cyclesÓ; similarly for other integers (including
both positive and negative; it doesnÕt change the graph) which the value of a
could be chosen, i.e., when a=n, the speed of the change of the value of
sin(at) is doubled, so the value of
sin(at) goes through n Òvibration cyclesÓ.
What
if we let x be sin(t), and change the values of c and
d? The rationale for this exploration would be similar as above. What if we
change the values of p and q? The point is that the graph would be either stretched
or squashed in two directions (x-axis and y-axis). There are still many other good cases we could try to
explore. We may find some other
interesting graphs. But I think all further explorations would be based on what
we have done fundamentally.
Return to my homepage for EMAT 6680